Equation Of Sphere In Standard Form

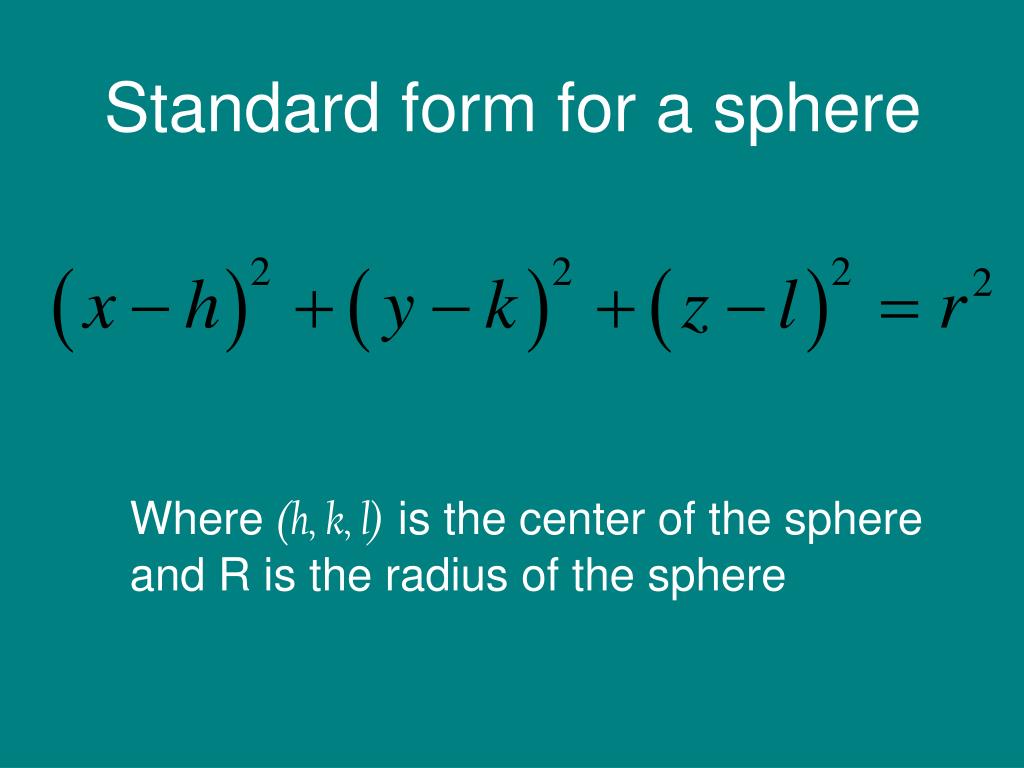
Equation Of Sphere In Standard Form - Which is called the equation of a sphere. Here, we are given the coordinates of the center of the sphere and, therefore, can deduce that 𝑎 = 1 1, 𝑏 = 8, and 𝑐 = − 5. Web answer we know that the standard form of the equation of a sphere is ( 𝑥 − 𝑎) + ( 𝑦 − 𝑏) + ( 𝑧 − 𝑐) = 𝑟, where ( 𝑎, 𝑏, 𝑐) is the center and 𝑟 is the length of the radius. Web the general formula is v 2 + a v = v 2 + a v + ( a / 2) 2 − ( a / 2) 2 = ( v + a / 2) 2 − a 2 / 4. Web what is the equation of a sphere in standard form? So we can use the formula of distance from p to c, that says: For z , since a = 2, we get z 2 + 2 z = ( z + 1) 2 − 1. (x −xc)2 + (y − yc)2 +(z −zc)2 = r2, Web now that we know the standard equation of a sphere, let's learn how it came to be: √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so:

√(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: Is the center of the sphere and ???r??? Web answer we know that the standard form of the equation of a sphere is ( 𝑥 − 𝑎) + ( 𝑦 − 𝑏) + ( 𝑧 − 𝑐) = 𝑟, where ( 𝑎, 𝑏, 𝑐) is the center and 𝑟 is the length of the radius. So we can use the formula of distance from p to c, that says: √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: Web the answer is: Points p (x,y,z) in the space whose distance from c(xc,yc,zc) is equal to r. Here, we are given the coordinates of the center of the sphere and, therefore, can deduce that 𝑎 = 1 1, 𝑏 = 8, and 𝑐 = − 5. In your case, there are two variable for which this needs to be done: Web what is the equation of a sphere in standard form?

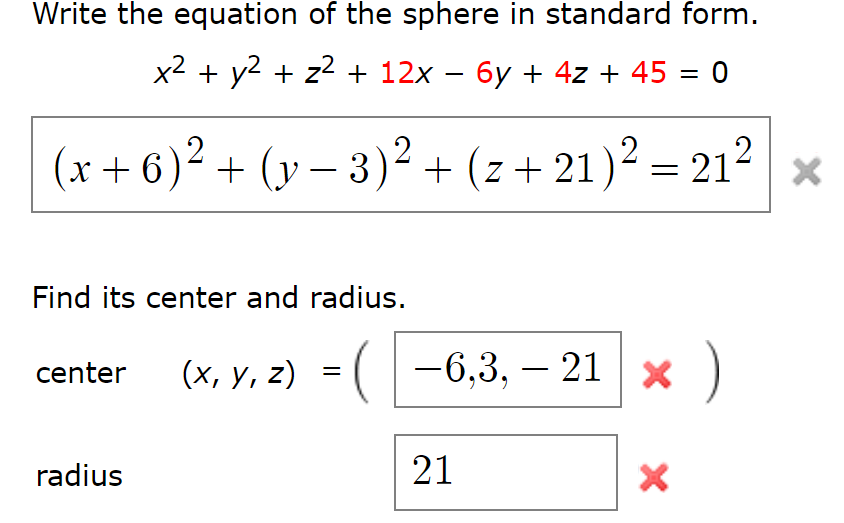
X2 + y2 +z2 + ax +by +cz + d = 0, this is because the sphere is the locus of all. Web express s t → s t → in component form and in standard unit form. For y , since a = − 4, we get y 2 − 4 y = ( y − 2) 2 − 4. Is the radius of the sphere. As described earlier, vectors in three dimensions behave in the same way as vectors in a plane. Web the formula for the equation of a sphere. Consider a point s ( x, y, z) s (x,y,z) s (x,y,z) that lies at a distance r r r from the center (. (x −xc)2 + (y − yc)2 +(z −zc)2 = r2, We are also told that 𝑟 = 3. Points p (x,y,z) in the space whose distance from c(xc,yc,zc) is equal to r.

PPT Equations of Spheres PowerPoint Presentation, free download ID

Consider a point s ( x, y, z) s (x,y,z) s (x,y,z) that lies at a distance r r r from the center (. We are also told that 𝑟 = 3. For y , since a = − 4, we get y 2 − 4 y = ( y − 2) 2 − 4. Web the formula for the.

Understanding Equation of a Sphere YouTube

So we can use the formula of distance from p to c, that says: √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: Is the radius of the sphere. √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: Consider a point s ( x, y, z) s (x,y,z) s (x,y,z) that lies.

Equation of the Sphere in Standard Form, Center, and Radius YouTube

Consider a point s ( x, y, z) s (x,y,z) s (x,y,z) that lies at a distance r r r from the center (. So we can use the formula of distance from p to c, that says: Web the answer is: As described earlier, vectors in three dimensions behave in the same way as vectors in a plane. To.

Solved Write the equation of the sphere in standard form. x2

So we can use the formula of distance from p to c, that says: Web now that we know the standard equation of a sphere, let's learn how it came to be: √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: Web the answer is: Web save 14k views 8 years ago calculus iii exam 1.

Equation of the Sphere in Standard Form, Center, and Radius Standard

Web the general formula is v 2 + a v = v 2 + a v + ( a / 2) 2 − ( a / 2) 2 = ( v + a / 2) 2 − a 2 / 4. Points p (x,y,z) in the space whose distance from c(xc,yc,zc) is equal to r. X2 + y2 +z2 +.

The principle of vector equation of a sphere Download Scientific Diagram

√(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: To calculate the radius of the sphere, we can use the distance formula Also learn how to identify the center of a sphere and the radius when given the equation of a sphere in standard. For y , since a = − 4, we get y 2.

Solved Write the equation of the sphere in standard form.

Is the center of the sphere and ???r??? Web the answer is: Web the formula for the equation of a sphere. Is the radius of the sphere. Also learn how to identify the center of a sphere and the radius when given the equation of a sphere in standard.

Equation, standard form, of a sphere iGCSE, Additional maths part 1

Also learn how to identify the center of a sphere and the radius when given the equation of a sphere in standard. Web answer we know that the standard form of the equation of a sphere is ( 𝑥 − 𝑎) + ( 𝑦 − 𝑏) + ( 𝑧 − 𝑐) = 𝑟, where ( 𝑎, 𝑏, 𝑐) is the.

Multivariable Calculus The equation of a sphere. YouTube

X2 + y2 +z2 + ax +by +cz + d = 0, this is because the sphere is the locus of all. In your case, there are two variable for which this needs to be done: Web answer we know that the standard form of the equation of a sphere is ( 𝑥 − 𝑎) + ( 𝑦 − 𝑏).

How can we Write the Equation of a Sphere in Standard Form? [Solved]

Web what is the equation of a sphere in standard form? As described earlier, vectors in three dimensions behave in the same way as vectors in a plane. Points p (x,y,z) in the space whose distance from c(xc,yc,zc) is equal to r. To calculate the radius of the sphere, we can use the distance formula Web x2 + y2 +.

So We Can Use The Formula Of Distance From P To C, That Says:

Web the general formula is v 2 + a v = v 2 + a v + ( a / 2) 2 − ( a / 2) 2 = ( v + a / 2) 2 − a 2 / 4. Web express s t → s t → in component form and in standard unit form. √(x −xc)2 + (y −yc)2 + (z − zc)2 = r and so: We are also told that 𝑟 = 3.

For Y , Since A = − 4, We Get Y 2 − 4 Y = ( Y − 2) 2 − 4.

If (a, b, c) is the centre of the sphere, r represents the radius, and x, y, and z are the coordinates of the points on the surface of the sphere, then the general equation of. So we can use the formula of distance from p to c, that says: Which is called the equation of a sphere. Web answer we know that the standard form of the equation of a sphere is ( 𝑥 − 𝑎) + ( 𝑦 − 𝑏) + ( 𝑧 − 𝑐) = 𝑟, where ( 𝑎, 𝑏, 𝑐) is the center and 𝑟 is the length of the radius.

(X −Xc)2 + (Y − Yc)2 +(Z −Zc)2 = R2,

In your case, there are two variable for which this needs to be done: To calculate the radius of the sphere, we can use the distance formula Here, we are given the coordinates of the center of the sphere and, therefore, can deduce that 𝑎 = 1 1, 𝑏 = 8, and 𝑐 = − 5. First thing to understand is that the equation of a sphere represents all the points lying equidistant from a center.

As Described Earlier, Vectors In Three Dimensions Behave In The Same Way As Vectors In A Plane.

Web x2 + y2 + z2 = r2. Is the center of the sphere and ???r??? X2 + y2 +z2 + ax +by +cz + d = 0, this is because the sphere is the locus of all. Also learn how to identify the center of a sphere and the radius when given the equation of a sphere in standard.