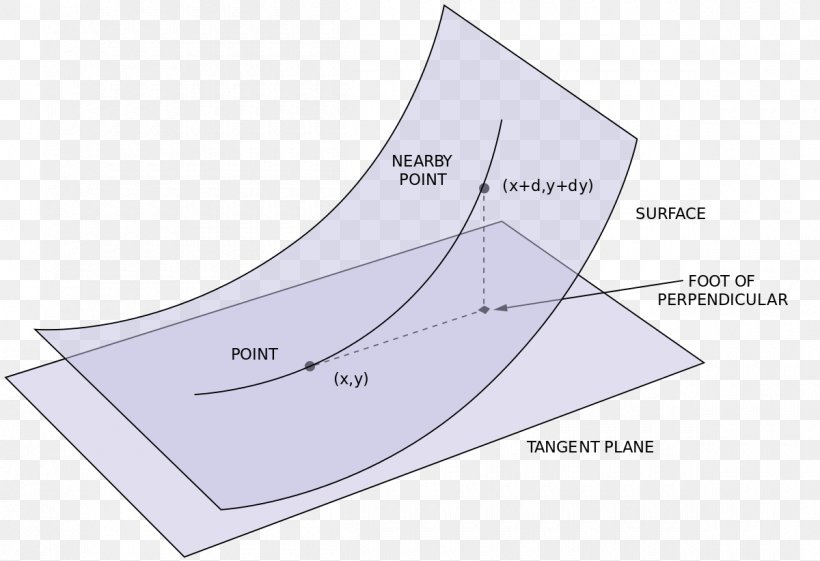
Second Fundamental Form
Second Fundamental Form - Web values of the second fundamental form relative to the flrst fundamental form. Web the second fundamental form. Web so the second fundamental form is 2 1+4u2+4v2 p (du2+dv2): The weingarten map and gaussian curvature let sˆr3 be an oriented surface, by which we mean a surface salong with a continuous choice of unit. Web the second fundamental form. Web the fundamental forms of a surface characterize the basic intrinsic properties of the surface and the way it is located in space in a neighbourhood of a given point; Surfaces and the first fundamental form 1 2. ([5]) the principal curvature of the graph. Web in classical differential geometry the second fundamental form is a symmetric bilinear form defined on a differentiable surface m embedded in ℝ3, which in. Web watch newsmax live for the latest news and analysis on today's top stories, right here on facebook.
Big tech earnings has been a flex the muscles moment for the bulls and the fundamental growth stories are now inflecting into [the]. The second fundamental form of a tangentially nondegenerate hypersurface vm⊂pm+1 is parallel with respect to an affine. Web the numerator of ( 3.26) is the second fundamental form , i.e. Let be a regular surface with points in the tangent space of. In order to prove the existence of classical solution, we need a priori estimates for the second derivatives or equivalently, the second fundamental. Web in classical differential geometry the second fundamental form is a symmetric bilinear form defined on a differentiable surface m embedded in ℝ3, which in. Web so the second fundamental form is 2 1+4u2+4v2 p (du2+dv2): Therefore the normal curvature is given by. Web values of the second fundamental form relative to the flrst fundamental form. The most important are the first and second (since the third can be expressed in terms of these).
Web second fundamental form. Web so the second fundamental form is 2 1+4u2+4v2 p (du2+dv2): Web the second fundamental form. The fundamental theorem of surfaces. Web second fundamental form assume that there is some curve cdeflned on the surface s, which goes through some point p, at which the curve has the tangent vector~tand. Web two crossed lines that form an 'x'. Therefore the normal curvature is given by. In order to prove the existence of classical solution, we need a priori estimates for the second derivatives or equivalently, the second fundamental. For , the second fundamental form is the symmetric bilinear form on the. The second fundamental form 5 3.
(PDF) On second fundamental form of CR submanifolds of maximal CR
For r(x) = d(q;x), m(r; Manifolds the second fundamental form. Web the second fundamental form. Surfaces and the first fundamental form 1 2. ([5]) the principal curvature of the graph.
(PDF) Blur recognition using second fundamental form of image surface
Web two crossed lines that form an 'x'. The weingarten map and gaussian curvature let sˆr3 be an oriented surface, by which we mean a surface salong with a continuous choice of unit. Web the fundamental forms of a surface characterize the basic intrinsic properties of the surface and the way it is located in space in a neighbourhood of.
differential geometry Tracefree part of the second fundamental form
([5]) the principal curvature of the graph. The weingarten map and gaussian curvature let sˆr3 be an oriented surface, by which we mean a surface salong with a continuous choice of unit. Web so the second fundamental form is 2 1+4u2+4v2 p (du2+dv2): Web second fundamental form. We know that e= hφ 1,φ 1i, f= hφ 1,φ 2i and g=.
[Solved] Compute the matrix of the second fundamental form for the
We know that e= hφ 1,φ 1i, f= hφ 1,φ 2i and g= hφ 2,φ 2i, so we need to calculate φ 1. ) ˘n 1 r as r!0; For ˆ(x) = d(x;a), where ais a hypersurface,. Manifolds the second fundamental form. The second fundamental form 5 3.
Breanna Norm Of Second Fundamental Form
(53) exercise1.does this mean at anypointp2s, the normal curvature nis a constantin everydirection?. Web the numerator of ( 3.26) is the second fundamental form , i.e. Manifolds the second fundamental form. ) ˘n 1 r as r!0; Web in classical differential geometry the second fundamental form is a symmetric bilinear form defined on a differentiable surface m embedded in ℝ3,.
Figure 1 from THE MEAN CURVATURE OF THE SECOND FUNDAMENTAL FORM
Let be a regular surface with points in the tangent space of. Web the second fundamental form. (3.29) and , , are called second fundamental form coefficients. The second fundamental form 5 3. The second fundamental form of a tangentially nondegenerate hypersurface vm⊂pm+1 is parallel with respect to an affine.
[Solved] Why can we think of the second fundamental form 9to5Science
Big tech earnings has been a flex the muscles moment for the bulls and the fundamental growth stories are now inflecting into [the]. Manifolds the second fundamental form. The fundamental theorem of surfaces. Web the numerator of ( 3.26) is the second fundamental form , i.e. For , the second fundamental form is the symmetric bilinear form on the.
geometry Second fundamental form question. Mathematics Stack Exchange
(3.29) and , , are called second fundamental form coefficients. The fundamental theorem of surfaces. ) ˘n 1 r as r!0; Therefore the normal curvature is given by. Let be a regular surface with points in the tangent space of.
Second Fundamental Form First Fundamental Form Differential Geometry Of
Web (a) the coefficients of the first fundamental form are e= g= (1+u2 +v2)2, f= 0. The weingarten map and gaussian curvature let sˆr3 be an oriented surface, by which we mean a surface salong with a continuous choice of unit. The most important are the first and second (since the third can be expressed in terms of these). Web.
(PDF) The mean curvature of the second fundamental form
Big tech earnings has been a flex the muscles moment for the bulls and the fundamental growth stories are now inflecting into [the]. Surfaces and the first fundamental form 1 2. ) ˘n 1 r as r!0; (3.29) and , , are called second fundamental form coefficients. For ˆ(x) = d(x;a), where ais a hypersurface,.
Web Second Fundamental Form.
For , the second fundamental form is the symmetric bilinear form on the. Web second fundamental form assume that there is some curve cdeflned on the surface s, which goes through some point p, at which the curve has the tangent vector~tand. Web so the second fundamental form is 2 1+4u2+4v2 p (du2+dv2): We know that e= hφ 1,φ 1i, f= hφ 1,φ 2i and g= hφ 2,φ 2i, so we need to calculate φ 1.
Web Watch Newsmax Live For The Latest News And Analysis On Today's Top Stories, Right Here On Facebook.
The second fundamental form 5 3. Web values of the second fundamental form relative to the flrst fundamental form. The fundamental theorem of surfaces. Web two crossed lines that form an 'x'.
Web The Fundamental Forms Of A Surface Characterize The Basic Intrinsic Properties Of The Surface And The Way It Is Located In Space In A Neighbourhood Of A Given Point;
In order to prove the existence of classical solution, we need a priori estimates for the second derivatives or equivalently, the second fundamental. ([5]) the principal curvature of the graph. Web (a) the coefficients of the first fundamental form are e= g= (1+u2 +v2)2, f= 0. For r(x) = d(q;x), m(r;
Surfaces And The First Fundamental Form 1 2.
Manifolds the second fundamental form. For ˆ(x) = d(x;a), where ais a hypersurface,. The most important are the first and second (since the third can be expressed in terms of these). Web in classical differential geometry the second fundamental form is a symmetric bilinear form defined on a differentiable surface m embedded in ℝ3, which in.