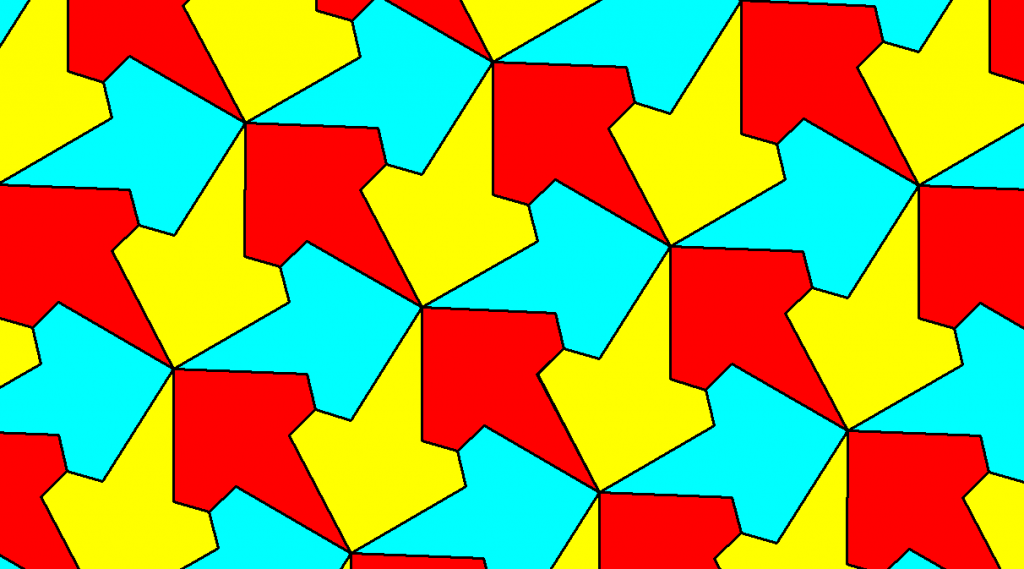
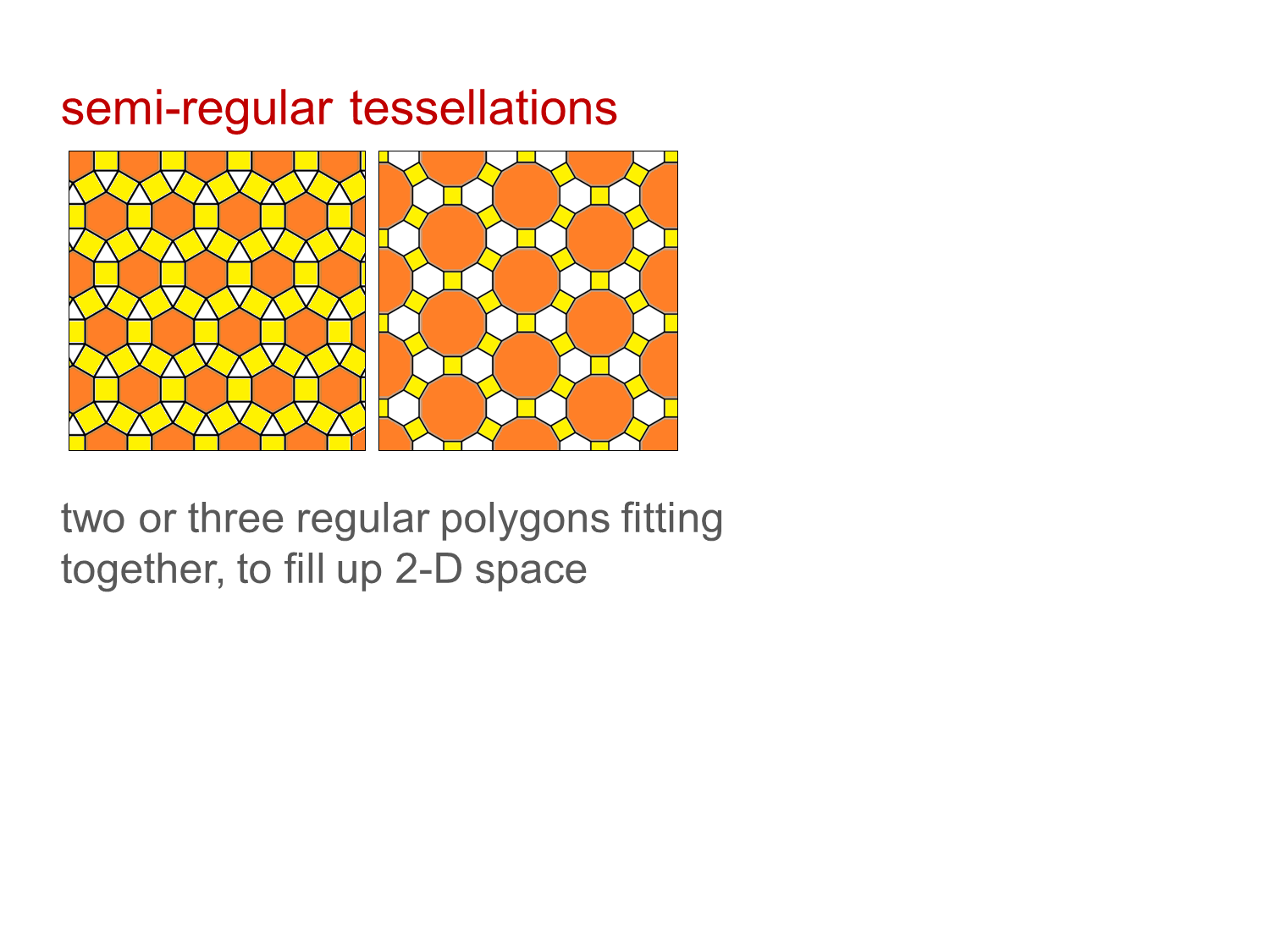
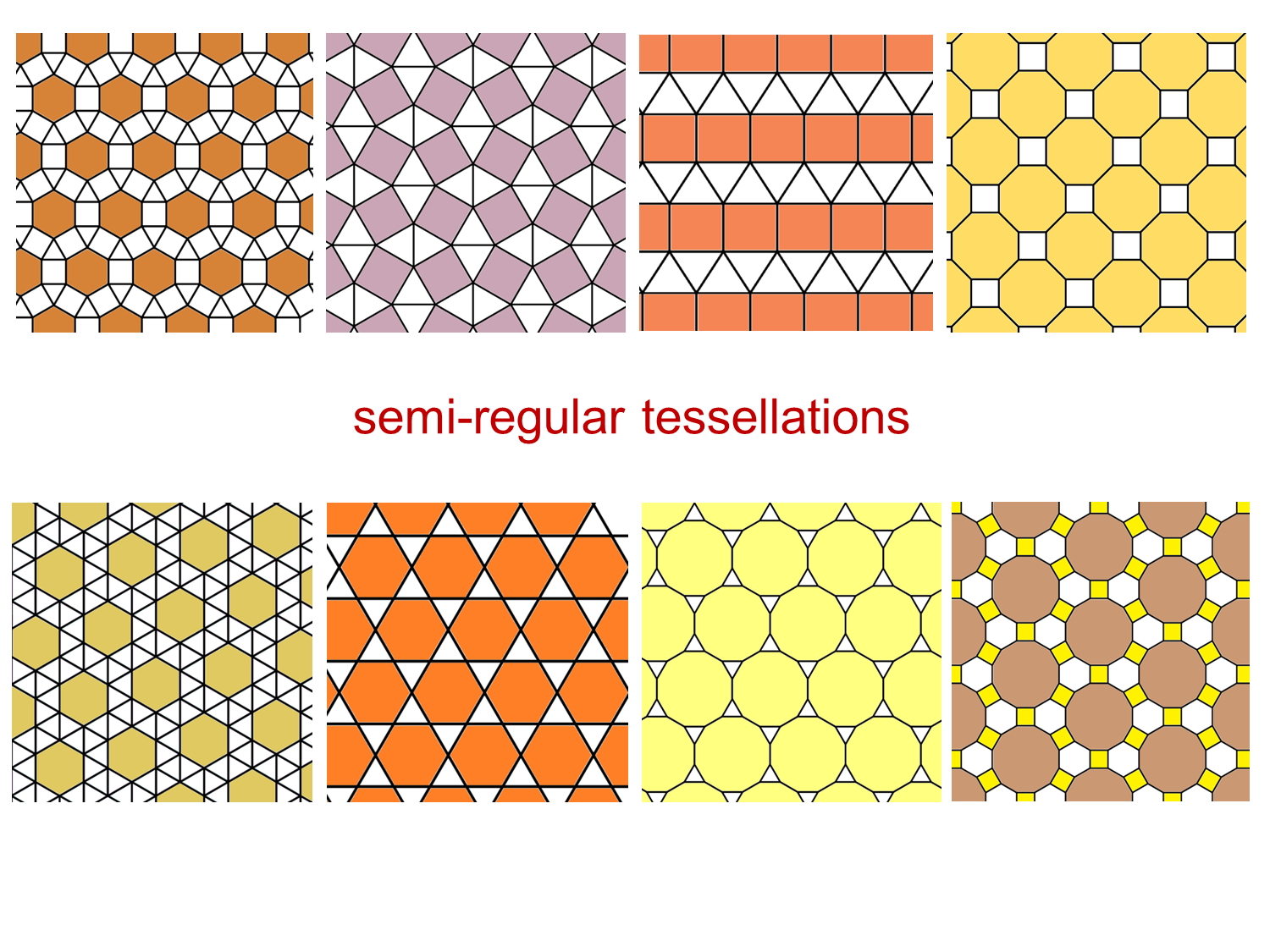
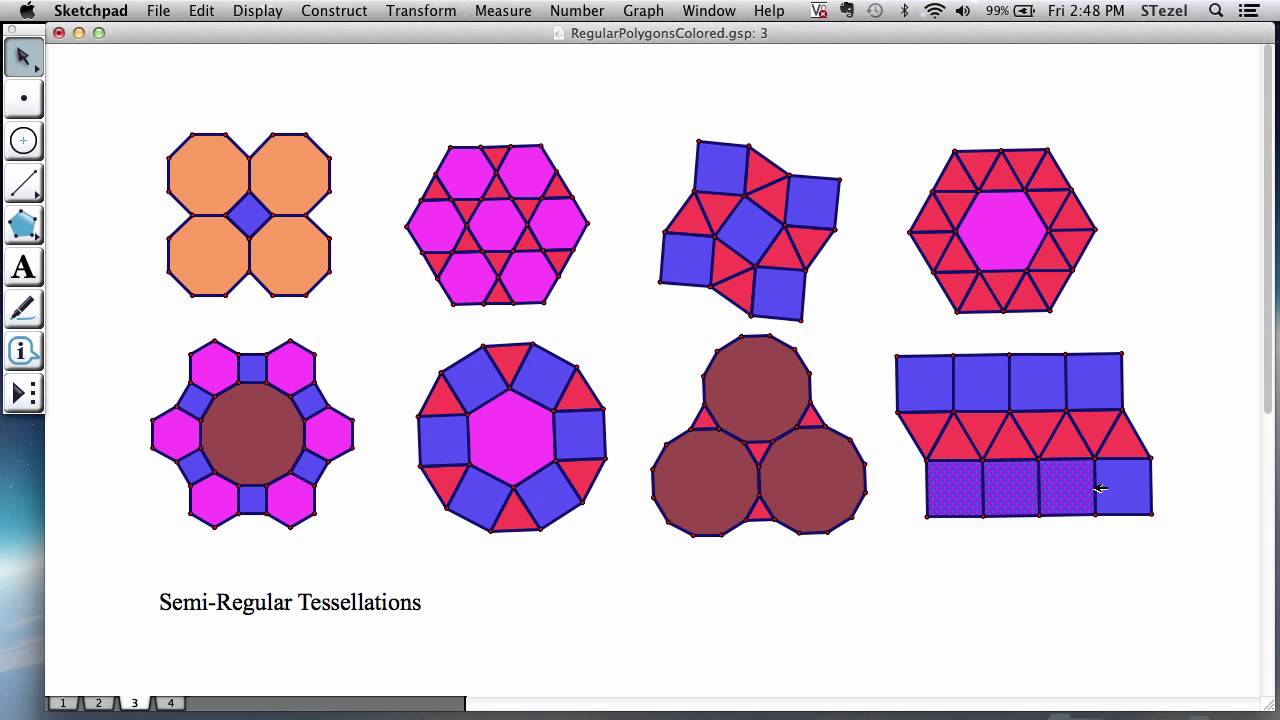
Which Of The Following Polygons Can Form A Regular Tessellation
Which Of The Following Polygons Can Form A Regular Tessellation - [6] [7] polygons in these meet at a point with no gap or overlap. Those made up of equilateral triangles, squares, or regular hexagons. Invite students to choose a corner point on the triangle tessellation. Web classifying tessellations there are 3 types of tessellations. Web other math questions and answers. Determine whether the given regular polygon(s) can be used to form a tessellation. Regular polygons tessellate if the interior angles can be added together to make 360°. Web there are only three regular tessellations: They are formed by two or more types of regular polygon, each with the same side length each. So, equilateral triangle cannot be used to.
They are formed by two or more types of regular polygon, each with the same side length each. Regular polygons tessellate if the interior angles can be added together to make 360°. Those made up of equilateral triangles, squares, or regular hexagons. It is only with these three shapes. Web classifying tessellations there are 3 types of tessellations. Regular tessellation a regular tessellation is made up of regular congruent polygons. Web there are only three regular tessellations: Web which of the following polygons can form a regular tessellation? So, equilateral triangle cannot be used to. Web a tessellation is a pattern created with identical shapes which fit together with no gaps.
Web there are only three regular tessellations: Web other math questions and answers. These types of tiling can be made either with regular. Web which of the following polygons can form a regular tessellation? Question 3 of 33 which of the following polygons can form a regular tessellation? Web the regular polygons that can be used to form a regular tessellation are an equilateral triangle, a square, and a regular hexagon. Web to form a regular tessellation, the angle measure of a regular polygon must be a divisor of 360 ∘ ^{\circ} ∘ in this case, regular polygon is octagon and each angle of octagon. Web classifying tessellations there are 3 types of tessellations. Web geometry geometry questions and answers which of the following polygons can form a regular tessellation? Web tessellations that are made with just one shape are called monohedral tiling, or regular tessellations.
Is there a 9 Sided Shape?
This means that the only regular polygons that tessellate are. Invite students to choose a corner point on the triangle tessellation. These types of tiling can be made either with regular. Regular polygons tessellate if the interior angles can be added together to make 360°. Web to form a regular tessellation, the angle measure of a regular polygon must be.
10.6 Video Investigation "Which Regular Polygons Tessellate?" YouTube
Web a regular tessellation is a design covering the plane made using 1 type of regular polygons. Determine whether the given regular polygon(s) can be used to form a tessellation. Web geometry geometry questions and answers which of the following polygons can form a regular tessellation? Web tessellations that are made with just one shape are called monohedral tiling, or.
Make maths more creative. Hollie Ferguson ePortfolio
Web tessellations that are made with just one shape are called monohedral tiling, or regular tessellations. Web check all that apply. Web classifying tessellations there are 3 types of tessellations. Regular tessellation a regular tessellation is made up of regular congruent polygons. Web to form a regular tessellation, the angle measure of a regular polygon must be a divisor of.
MEDIAN Don Steward mathematics teaching semi regular tessellations
Invite students to choose a corner point on the triangle tessellation. It is only with these three shapes. Web other math questions and answers. Web a tessellation is a pattern created with identical shapes which fit together with no gaps. Web a regular tessellation is a design covering the plane made using 1 type of regular polygons.
Actv regular tessellations
Determine whether the given regular polygon(s) can be used to form a tessellation. So, equilateral triangle cannot be used to. These types of tiling can be made either with regular. [6] [7] polygons in these meet at a point with no gap or overlap. Web geometry geometry questions and answers which of the following polygons can form a regular tessellation?
MEDIAN Don Steward mathematics teaching semi regular tessellations
Regular tessellation a regular tessellation is made up of regular congruent polygons. Regular polygons tessellate if the interior angles can be added together to make 360°. Web classifying tessellations there are 3 types of tessellations. It is only with these three shapes. Web the regular polygons that can be used to form a regular tessellation are an equilateral triangle, a.
Regular Polygons in a Tessellation Triangles, Squares, Hexagons, and
Web geometry geometry questions and answers which of the following polygons can form a regular tessellation? Web which of the following polygons can form a regular tessellation? Web a regular tessellation is a design covering the plane made using 1 type of regular polygons. Web you can similarly check that, just like pentagons, any regular polygon with 7 or more.
Tessellations (4) SemiRegular Tessellations YouTube
These types of tiling can be made either with regular. Web classifying tessellations there are 3 types of tessellations. Web the regular polygons that can be used to form a regular tessellation are an equilateral triangle, a square, and a regular hexagon. Question 3 of 33 which of the following polygons can form a regular tessellation? Web which of the.
Tessellations (2) Regular Polygon Construction YouTube
Web here is a table to prove why there are only three regular polygons that create regular tessellations: Web the regular polygons that can be used to form a regular tessellation are an equilateral triangle, a square, and a regular hexagon. Question 3 of 33 which of the following polygons can form a regular tessellation? Web geometry geometry questions and.
Which of the following polygons can form a regular tessellation
Invite students to choose a corner point on the triangle tessellation. Web tessellations that are made with just one shape are called monohedral tiling, or regular tessellations. Web there are only three regular tessellations: Web which of the following polygons can form a regular tessellation? Regular polygons tessellate if the interior angles can be added together to make 360°.
Web Here Is A Table To Prove Why There Are Only Three Regular Polygons That Create Regular Tessellations:
Web the regular polygons that can be used to form a regular tessellation are an equilateral triangle, a square, and a regular hexagon. So, equilateral triangle cannot be used to. Web check all that apply. Regular polygons tessellate if the interior angles can be added together to make 360°.
Web Other Math Questions And Answers.
Web geometry geometry questions and answers which of the following polygons can form a regular tessellation? Web classifying tessellations there are 3 types of tessellations. Web tessellations that are made with just one shape are called monohedral tiling, or regular tessellations. Web you can similarly check that, just like pentagons, any regular polygon with 7 or more sides doesn’t tessellate.
Web To Form A Regular Tessellation, The Angle Measure Of A Regular Polygon Must Be A Divisor Of 360 ∘ ^{\Circ} ∘ In This Case, Regular Polygon Is Octagon And Each Angle Of Octagon.
Determine whether the given regular polygon(s) can be used to form a tessellation. These types of tiling can be made either with regular. Those made up of equilateral triangles, squares, or regular hexagons. Regular tessellation a regular tessellation is made up of regular congruent polygons.
Determine Whether The Given Regular Polygon(S) Can Be Used To Form A Tessellation.
Invite students to choose a corner point on the triangle tessellation. This means that the only regular polygons that tessellate are. Web there are only three regular tessellations: It is only with these three shapes.