Maxwell Equation In Differential Form
Maxwell Equation In Differential Form - Maxwell was the first person to calculate the speed of propagation of electromagnetic waves, which was the same as the speed of light and came to the conclusion that em waves and visible light are similar. (note that while knowledge of differential equations is helpful here, a conceptual understanding is possible even without it.) gauss’ law for electricity differential form: This paper begins with a brief review of the maxwell equationsin their \di erential form (not to be confused with the maxwell equationswritten using the language of di erential forms, which we will derive in thispaper). Web what is the differential and integral equation form of maxwell's equations? Web maxwell’s first equation in integral form is. Web we shall derive maxwell’s equations in differential form by applying maxwell’s equations in integral form to infinitesimal closed paths, surfaces, and volumes, in the limit that they shrink to points. Web the differential form of maxwell’s equations (equations 9.1.3, 9.1.4, 9.1.5, and 9.1.6) involve operations on the phasor representations of the physical quantities. Web the differential form of maxwell’s equations (equations 9.1.10, 9.1.17, 9.1.18, and 9.1.19) involve operations on the phasor representations of the physical quantities. These equations have the advantage that differentiation with respect to time is replaced by multiplication by. Web the simplest representation of maxwell’s equations is in differential form, which leads directly to waves;
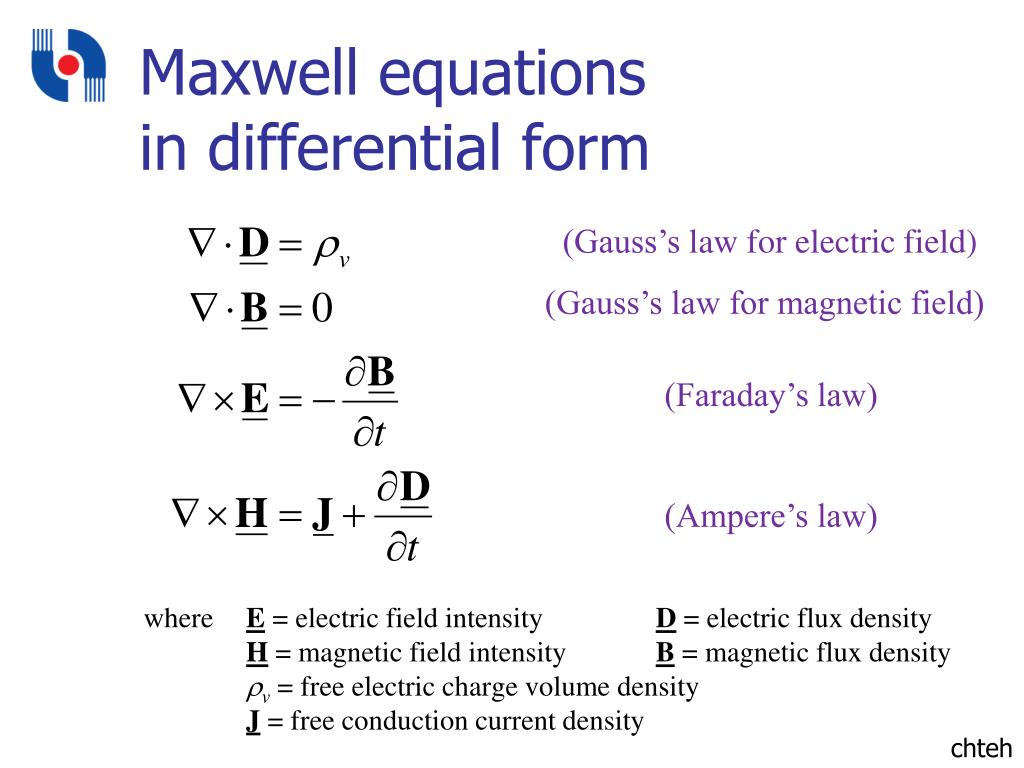
The del operator, defined in the last equation above, was seen earlier in the relationship between the electric field and the electrostatic potential. ∫e.da =1/ε 0 ∫ρdv, where 10 is considered the constant of proportionality. Maxwell was the first person to calculate the speed of propagation of electromagnetic waves, which was the same as the speed of light and came to the conclusion that em waves and visible light are similar. The differential form of this equation by maxwell is. Web maxwell’s equations in differential form ∇ × ∇ × ∂ b = − − m = − m − ∂ t mi = j + j + ∂ d = ji c + j + ∂ t jd ∇ ⋅ d = ρ ev ∇ ⋅ b = ρ mv ∂ = b , ∂ d ∂ jd t = ∂ t ≡ e electric field intensity [v/m] ≡ b magnetic flux density [weber/m2 = v s/m2 = tesla] ≡ m impressed (source) magnetic current density [v/m2] m ≡ Web maxwell’s equations are the basic equations of electromagnetism which are a collection of gauss’s law for electricity, gauss’s law for magnetism, faraday’s law of electromagnetic induction, and ampere’s law for currents in conductors. Web differentialform ∙ = or ∙ = 0 gauss’s law (4) × = + or × = 0 + 00 ampère’s law together with the lorentz force these equationsform the basic of the classic electromagnetism=(+v × ) ρ= electric charge density (as/m3) =0j= electric current density (a/m2)0=permittivity of free space lorentz force Differential form with magnetic and/or polarizable media: This equation was quite revolutionary at the time it was first discovered as it revealed that electricity and magnetism are much more closely related than we thought. So these are the differential forms of the maxwell’s equations.
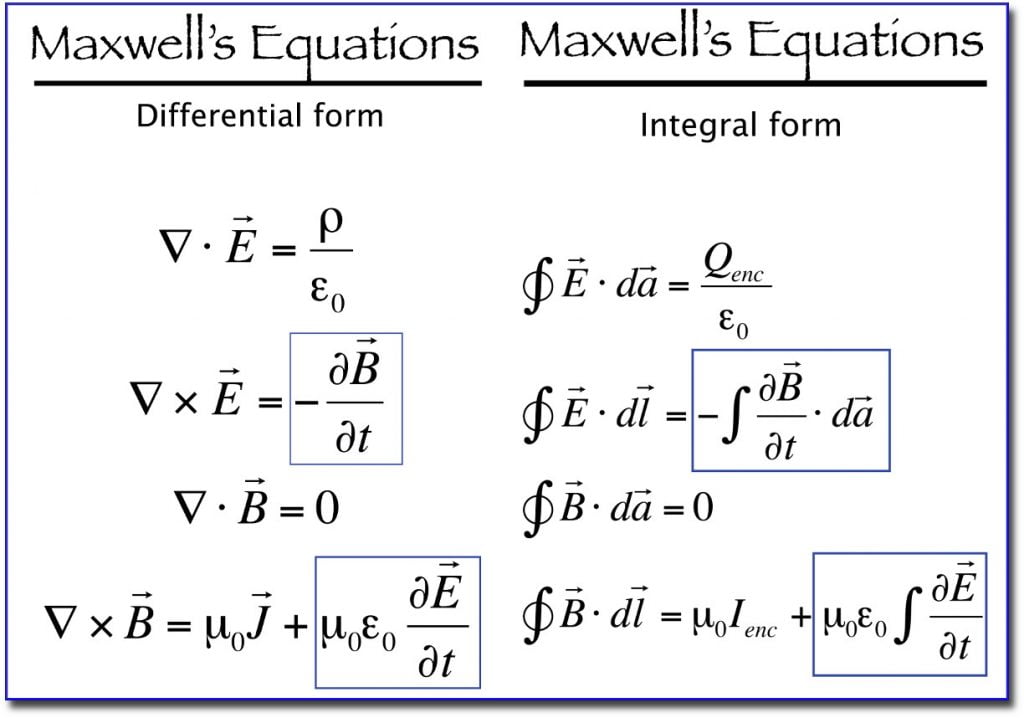
In order to know what is going on at a point, you only need to know what is going on near that point. \bm {∇∙e} = \frac {ρ} {ε_0} integral form: The differential form of this equation by maxwell is. Web the differential form of maxwell’s equations (equations 9.1.10, 9.1.17, 9.1.18, and 9.1.19) involve operations on the phasor representations of the physical quantities. From them one can develop most of the working relationships in the field. The differential form uses the overlinetor del operator ∇: Maxwell’s second equation in its integral form is. Web maxwell’s equations are the basic equations of electromagnetism which are a collection of gauss’s law for electricity, gauss’s law for magnetism, faraday’s law of electromagnetic induction, and ampere’s law for currents in conductors. ∇ ⋅ e = ρ / ϵ0 ∇ ⋅ b = 0 ∇ × e = − ∂b ∂t ∇ × b = μ0j + 1 c2∂e ∂t. The del operator, defined in the last equation above, was seen earlier in the relationship between the electric field and the electrostatic potential.
Maxwell’s Equations Equivalent Currents Maxwell’s Equations in Integral
(2.4.12) ∇ × e ¯ = − ∂ b ¯ ∂ t applying stokes’ theorem (2.4.11) to the curved surface a bounded by the contour c, we obtain: In that case, the del operator acting on a scalar (the electrostatic potential), yielded a vector quantity (the electric field). Maxwell's equations represent one of the most elegant and concise ways to.
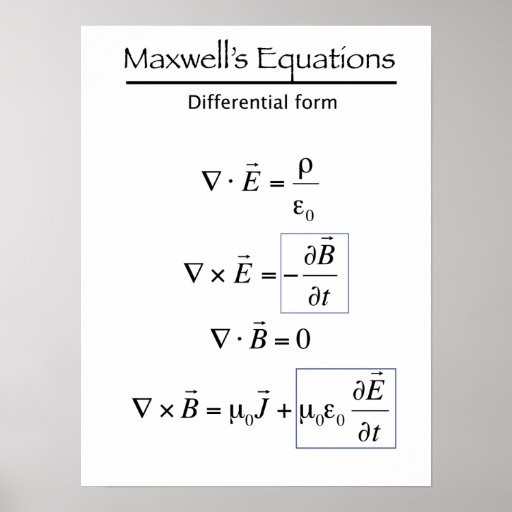
maxwells_equations_differential_form_poster
The alternate integral form is presented in section 2.4.3. The differential form uses the overlinetor del operator ∇: These equations have the advantage that differentiation with respect to time is replaced by multiplication by. Rs b = j + @te; In that case, the del operator acting on a scalar (the electrostatic potential), yielded a vector quantity (the electric field).
Maxwell's 4th equation derivation YouTube
Web in differential form, there are actually eight maxwells's equations! Web maxwell’s equations in differential form ∇ × ∇ × ∂ b = − − m = − m − ∂ t mi = j + j + ∂ d = ji c + j + ∂ t jd ∇ ⋅ d = ρ ev ∇ ⋅ b = ρ.
Maxwells Equations Differential Form Poster Zazzle
Rs + @tb = 0; Web maxwell’s equations are the basic equations of electromagnetism which are a collection of gauss’s law for electricity, gauss’s law for magnetism, faraday’s law of electromagnetic induction, and ampere’s law for currents in conductors. Web maxwell’s equations in differential form ∇ × ∇ × ∂ b = − − m = − m − ∂.
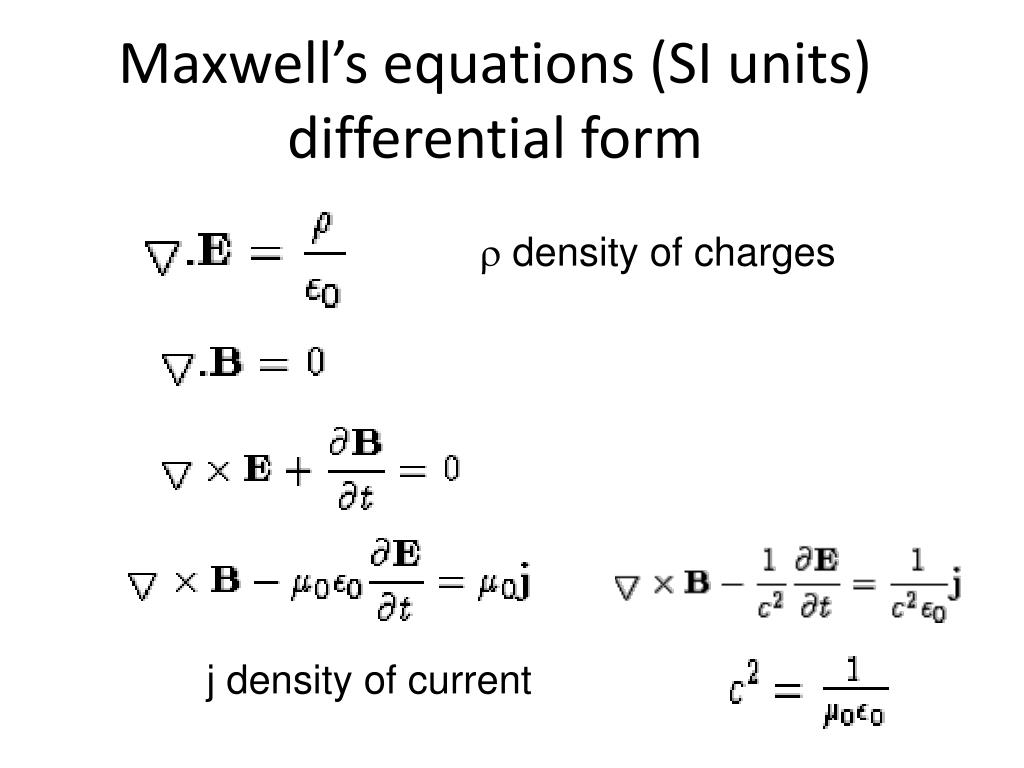
PPT Maxwell’s equations PowerPoint Presentation, free download ID
In order to know what is going on at a point, you only need to know what is going on near that point. Web the differential form of maxwell’s equations (equations 9.1.10, 9.1.17, 9.1.18, and 9.1.19) involve operations on the phasor representations of the physical quantities. Web maxwell’s equations in differential form ∇ × ∇ × ∂ b = −.
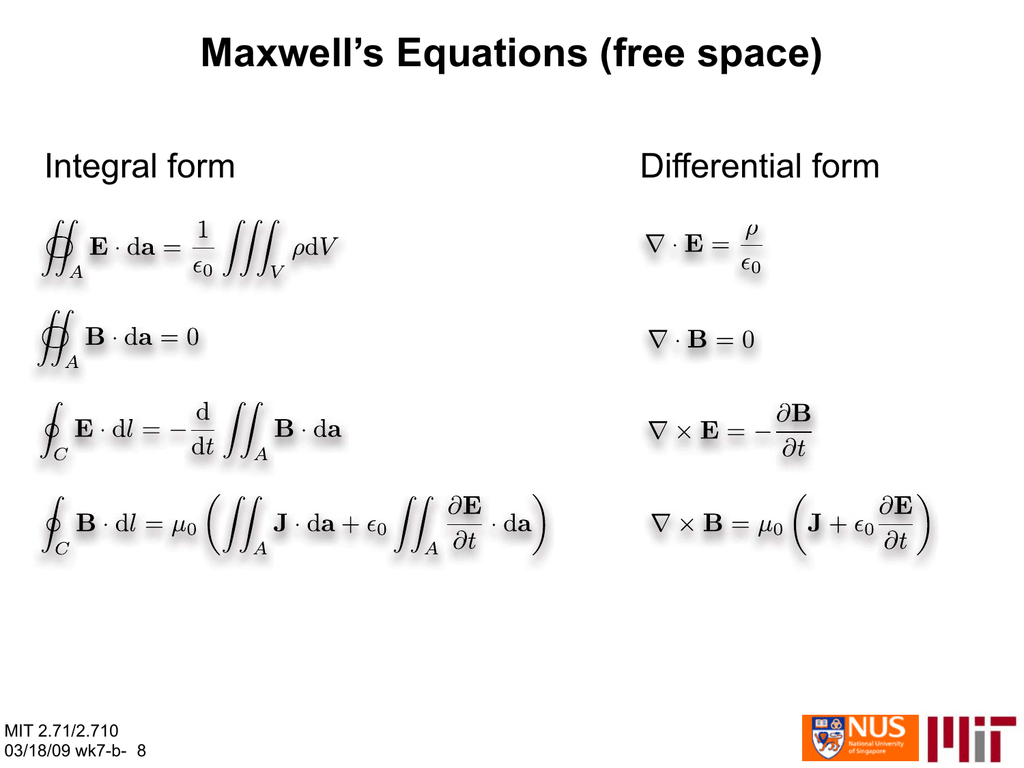
Maxwell’s Equations (free space) Integral form Differential form MIT 2.
Differential form with magnetic and/or polarizable media: ∫e.da =1/ε 0 ∫ρdv, where 10 is considered the constant of proportionality. Web answer (1 of 5): The del operator, defined in the last equation above, was seen earlier in the relationship between the electric field and the electrostatic potential. The differential form uses the overlinetor del operator ∇:
think one step more.. July 2011
There are no magnetic monopoles. Web maxwell’s equations are the basic equations of electromagnetism which are a collection of gauss’s law for electricity, gauss’s law for magnetism, faraday’s law of electromagnetic induction, and ampere’s law for currents in conductors. Its sign) by the lorentzian. Web the classical maxwell equations on open sets u in x = s r are as.
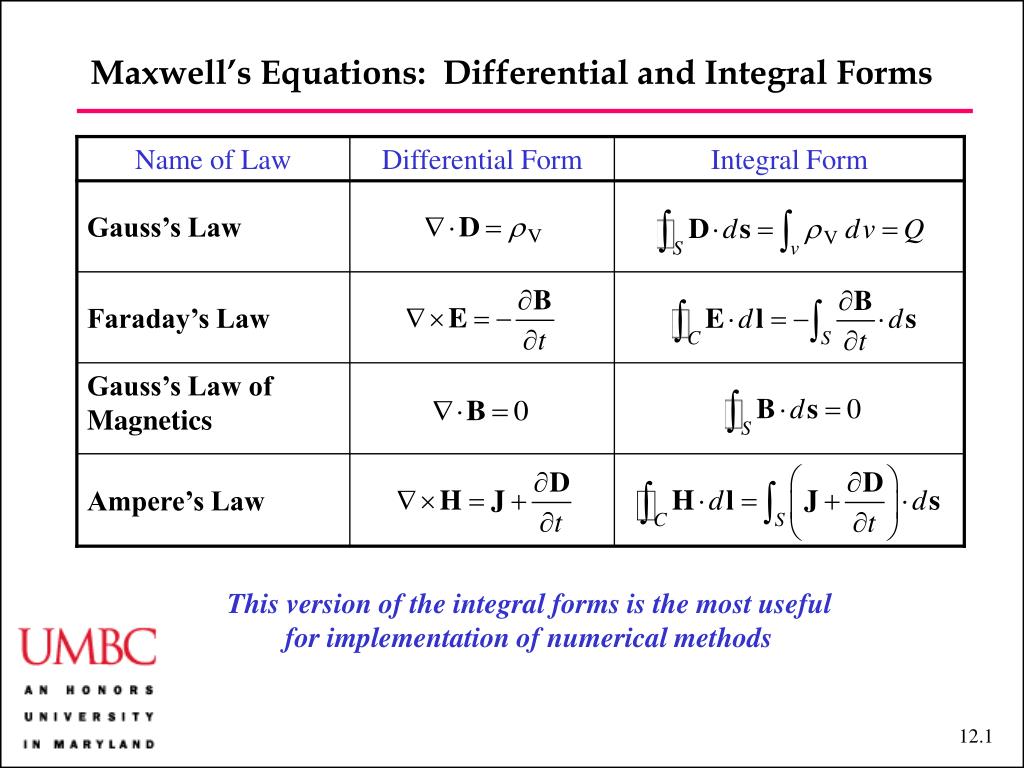
PPT Maxwell’s Equations Differential and Integral Forms PowerPoint
(note that while knowledge of differential equations is helpful here, a conceptual understanding is possible even without it.) gauss’ law for electricity differential form: Maxwell’s second equation in its integral form is. So these are the differential forms of the maxwell’s equations. Web we shall derive maxwell’s equations in differential form by applying maxwell’s equations in integral form to infinitesimal.
PPT EMF2016 THEORY PowerPoint Presentation, free
Maxwell's equations in their integral. Web the simplest representation of maxwell’s equations is in differential form, which leads directly to waves; There are no magnetic monopoles. Maxwell’s second equation in its integral form is. These equations have the advantage that differentiation with respect to time is replaced by multiplication by.
Fragments of energy, not waves or particles, may be the fundamental
Web differential forms and their application tomaxwell's equations alex eastman abstract. Web answer (1 of 5): Maxwell's equations in their integral. So these are the differential forms of the maxwell’s equations. Web the differential form of maxwell’s equations (equations 9.1.10, 9.1.17, 9.1.18, and 9.1.19) involve operations on the phasor representations of the physical quantities.
Its Sign) By The Lorentzian.
So these are the differential forms of the maxwell’s equations. The differential form uses the overlinetor del operator ∇: Web maxwell’s equations maxwell’s equations are as follows, in both the differential form and the integral form. Web maxwell’s equations in differential form ∇ × ∇ × ∂ b = − − m = − m − ∂ t mi = j + j + ∂ d = ji c + j + ∂ t jd ∇ ⋅ d = ρ ev ∇ ⋅ b = ρ mv ∂ = b , ∂ d ∂ jd t = ∂ t ≡ e electric field intensity [v/m] ≡ b magnetic flux density [weber/m2 = v s/m2 = tesla] ≡ m impressed (source) magnetic current density [v/m2] m ≡
(Note That While Knowledge Of Differential Equations Is Helpful Here, A Conceptual Understanding Is Possible Even Without It.) Gauss’ Law For Electricity Differential Form:
\bm {∇∙e} = \frac {ρ} {ε_0} integral form: Rs e = where : In that case, the del operator acting on a scalar (the electrostatic potential), yielded a vector quantity (the electric field). The electric flux across a closed surface is proportional to the charge enclosed.
Web In Differential Form, There Are Actually Eight Maxwells's Equations!
∂ j = h ∇ × + d ∂ t ∂ = − ∇ × e b ∂ ρ = d ∇ ⋅ t b ∇ ⋅ = 0 few other fundamental relationships j = σe ∂ ρ ∇ ⋅ j = − ∂ t d = ε e b = μ h ohm' s law continuity equation constituti ve relationsh ips here ε = ε ε (permittiv ity) and μ 0 = μ This paper begins with a brief review of the maxwell equationsin their \di erential form (not to be confused with the maxwell equationswritten using the language of di erential forms, which we will derive in thispaper). Web the simplest representation of maxwell’s equations is in differential form, which leads directly to waves; Electric charges produce an electric field.
There Are No Magnetic Monopoles.
Rs + @tb = 0; Web maxwell's equations are a set of four differential equations that form the theoretical basis for describing classical electromagnetism: Maxwell's equations represent one of the most elegant and concise ways to state the fundamentals of electricity and magnetism. ∇ ⋅ e = ρ / ϵ0 ∇ ⋅ b = 0 ∇ × e = − ∂b ∂t ∇ × b = μ0j + 1 c2∂e ∂t.